Let X and Y be two continuous random variables with joint probability density f(x, y). The joint

Question:

Let X and Y be two continuous random variables with joint probability density f(x, y). The joint distribution function F(a, b) is defined as follows:

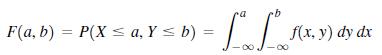
Verify each of the following:

a. F (-∞, -∞) = F(-∞, y) = F(x, -∞) = 0

b. F (∞, ∞) = 1

c. If a₂≥ a₁ and b₂ ≥ b₁ ,then F (a₂, b₂) - F (a₁, b₂) ≥ F (a₂, b₁) - F (a₁, b₁).

Fantastic news! We've Found the answer you've been seeking!

Step by Step Answer:

Related Book For answer-question

Statistics For Engineering And The Sciences

ISBN: 9781498728850

6th Edition

Authors: William M. Mendenhall, Terry L. Sincich

See More Books

Question Posted: Apr 14, 2021 07:30 AM

See More Questions

Let X and Y be two continuous random variables with joint density f(x, y). Show that f 2( y x)f 1 (x) = f 1 (x y)f 2 (y)
Let X and Y be two continuous random variables defined over the unit square. What does c equal if fX,Y (x, y)=c(x2 + y2)?
Let X and Y be independent continuous random variables with respective hazard rate functions X(t) and Y(t), and set W = min(X,Y). (a) Determine the distribution function of W in terms of those of X...
The two tugboats each exert a constant force F on the boat such that these forces are always directed perpendicular to the boats centerline. If the boat has a mass m and a radius of gyration k G...
1. Why do nonprofit organizations such as the San Diego Zoological Society need an appraisal system if their goal is not to maximize their profits? 2. What do you think are the pros and cons of using...
Using a financial calculator or spreadsheet, calculate the future value in 2 years of $10,000 invested today in an account that pays a stated annual interest rate of 12%, compounded monthly.
At 3:00 a.m. on November 22, 2010, 16-year-old Sydney McLemore was driving a Mazda3 with her friend, Natalie Hurst, in the front passenger seat. The vehicle was traveling south on Ross Bridge Highway...
Fabulator, Inc. produces and sells fashion clothing. On July 1, 2012, Fabulator, Inc. issued $120,000,000 of 20-year, 14% bonds at a market (effective) interest rate of 11%, receiving cash of...
6. [5] (A) Use integration to solve the "differential equation", dy dx 4 1+x' Note that the value of the solution at x=1 is . y(0) = 0 (B) Get a numerical approximation to 7 by using Euler's Method...
Managing Scope Changes Case Study Scope changes on a project can occur regardless of how well the project is planned or executed. Scope changes can be the result of something that was omitted during...
Refer to the IEEE Transactions on Information Forensics and Security (March 2013) study of wireless identify theft using cloned credit or debit cards, Exercise 6.3. On average, how many genuine balls...
Let X and Y be two continuous random variables with joint probability density a. Find the value of c. b. Find f 1 (x). c. Find f 2 (y). d. Find f 1 (x y). e. Find f 2 (x y). f. Find P (X 1 and Y ...
In Example 2, what percentage of the test scores are between 350 and 650? Data from Example 2 The scores on a certain achievement test are normally distributed with a mean of 500 and a standard...
Consider the circuit shown below. v(t) R C Here R = 3 KQ, C = 6 nF, and 1=1 mH. The voltage source is vt) (12 V) sin(w), where w=39 krad/s. = The current through the source is (t) = f sin(wt+0),...
If f(x) = f'(x) = x - 3 x +3 3x (x + 3) f'(3) = 33 (3+3) find:
Suppose the current yield on a one-year zero-coupon bond is 4 %, while the yield on a five-year zero-coupon bond is 6 %. Neither bond has any risk of default. Suppose you plan to invest for one year....
To analyze a stock, you have estimated the single-index model: ri - rf = alpha + beta x (ri - rm) + ei, for the stock using a market index portfolio. The following table summarizes the model...
Find the value for k so that the function will be continuous at x = -5. f(x) 5x+45x + 100 x+5 -7 3x2 12x + 12+ k if x < -5 if x -5
Show how each of the following compounds can be prepared from the given starting material. In each case, you will need to use a protecting group. a. b. c. CH3 CH,CHCH,CCH, CH,CHCH-COCH3 - OH OH Cl...
Consider the sections of two circuits illustrated above. Select True or False for all statements.After connecting a and b to a battery, the voltage across R1 always equals the voltage across R2.Rcd...
Round each number as indicated: (a) 1.236 7 to 4 significant figures (b) 1.238 4 to 4 significant figures (c) 0.135 2 to 3 significant figures (d) 2.051 to 2 significant figures (e) 2.005 0 to 3...
Round each number to three significant figures: (a) 0.216 74 (b) 0.216 5 (c) 0.216 500 3
Vernier scale. The figure below shows a scale found on instruments such as a micrometer caliper used for accurately measuring dimensions of objects. The lower scale slides along the upper scale and...
). BaTiCs (Ba+Ti4+02-) exhibits the ionic displacements at room temperature illustrated in Fig (1). The lattice constants are c = 4.036 and a = 3.992 . Calculate the magnitude of the spontaneous...
Describe a quantitative research project you would be interested in starting now or in the near future. Explain the problem and why this would be important for you to study? Why is a quantitative...
6.1 (a) Given a small-angle tilt boundary whose angle of tilt is 0.1, find the spacing between the dislocations in the boundary if the Burgers vector of the dislocations is 0.33 nm. (b) On the...

Let X and Y be two continuous random variables with joint probability density f(x, y). The joint

Question:

F(a, b) = P(X s a, Y s b) = f(x, y) dy dx %3D

Step by Step Answer:

To verify each of the statements we will use the properties of the joint probability density function PDF and the cumulative distribution function CDF ...View the full answer

Statistics For Engineering And The Sciences

Students also viewed these Engineering questions