Question: (a) Test the hypothesis that (mu_{1} eq mu_{2}) at the (alpha=0.1) level of significance for the given sample data. (b) Construct a (90 %) confidence

(a) Test the hypothesis that \(\mu_{1} eq \mu_{2}\) at the \(\alpha=0.1\) level of significance for the given sample data.

(b) Construct a \(90 \%\) confidence interval for \(\mu_{1}-\mu_{2}\).

(c) Test the hypothesis that \(\sigma_{1} eq \sigma_{2}\) at the \(\alpha=0.05\) level of significance for the given sample data.

n IK Sample 1 13 32.4 Sample 2 8 28.2 S 4.5

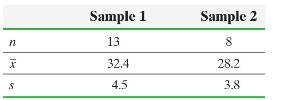
Assume that the populations are normally distributed and that independent sampling occurred.

n IK Sample 1 13 32.4 Sample 2 8 28.2 S 4.5 3.8

Step by Step Solution

★★★★★

3.41 Rating (151 Votes )

There are 3 Steps involved in it

1 Expert Approved Answer
Step: 1 Unlock

To perform these tasks well conduct both hypothesis tests and construct a confidence interval based ... View full answer

blur-text-image
Question Has Been Solved by an Expert!

Get step-by-step solutions from verified subject matter experts

Step: 2 Unlock
Step: 3 Unlock

Students Have Also Explored These Related Statistics Informed Decisions Using Data Questions!

Q:

(a) Test the hypothesis that \(\mu_{1} eq \mu_{2}\) at the \(\alpha=0.1\) level of significance for the given sample data. (b) Construct a 95\% confidence interval for \(\mu_{1}-\mu_{2}\). (c) Test...

Q:

Test the hypothesis that µ1 > µ2 at the α = 0.01 level of signiï¬cance for the given sample data. Assume that the populations are normally distributed and that...

Q:

The following samples are observations taken from the same elements at two different times: a. Assume that the populations are normally distributed and construct a 90% confidence interval for the...

Q:

1. Rhino viruses typically cause common colds. In a test of the effectiveness of echinacea, 32 of rhe 46 subjects treared with echinacea developed rhinovirus infections. In a placebo group, 44 of the...

Q:

Rhino viruses typically cause common colds. In a test of the effectiveness of echinacea, 32 of rhe 41 subjects treared with echinacea developed rhinovirus infections. In a placebo group, 55 of the 78...

Q:

B) Inferential Statistics 1. Since you are working with a small sample (fewer than 30 data values). construct a normal probability plot or quantile-quantile (QQ) plot to assess normality. Use this...

Q:

we have two simple random samples of leatherback turtles from the Pacific Ocean and from the Atlantic Ocean . the average weights from each sample were recorded in pounds. Danielles boss wants her to...

Q:

Hey help me solve this please 3) A recent national study found that 27% of college students are raising children. The administration of a local college found that of the 552 newly enrolled students,...

Q:

Practice problems: Consider a randomly selected group of 100 students. Suppose 40 students are majoring in biology or chemistry. Suppose 15 students major chemistry. Assume that 5 students are...

Q:

Score: Week 4 Confidence Intervals and Chi Square (Chs 11 - 12) For questions 3 and 4 below, be sure to list the null and alternate hypothesis statements. Use .05 for your significance...

Q:

Write a short summary describing your business idea. Before implementing an idea, you should test for its feasibility. The goal of a feasibility study is to determine whether to pursue an idea, wait...

Q:

Your mom decided to join their office cooperative (an organization owned and run by bits members who share profits and benefits) and agreed to contribute P 1,000 per month beginning in January 2019...

Q:

A contractor estimates maintenance costs for a new piece of equipment to be $ 2 7 5 for the first month with a monthly increase of 0 . 5 % . At i = 0 . 7 5 % per month, what is the present cost of...

Q:

Define the outputs or services provided by the program or departmental area.

Q:

Find the exact value, if any, of each composite function. If there is no value, say it is not defined. Do not use a calculator. tan -1 [tan(2/3)]

Q:

Find the exact value, if any, of each composite function. If there is no value, say it is not defined. Do not use a calculator. sin -1 [sin(-/8)]

Q:

Find the exact value, if any, of each composite function. If there is no value, say it is not defined. Do not use a calculator. cos -1 [cos(15/7)]

Q:

12. Effects of Inflation. Look at Table 10.1 and Figure 10.7 in the text. When were T-bill rates at their highest over the period from 1926 through 2014? Why do you think they were so high during...

Q:

What is meant by demixing? Describe the types of demixing that can occur?

Q:

what would 1 0 0 0 be written as in big O notation