A manufacturer of breakfast cereals has been accused of systematically underfilling their cereal packages. The manufacturer claims that the complaint must stem from settling of the cereal that occurs in shipment, and that the air space at the top of the package is normal settling of the contents. They claim that the filling process is normally distributed, and that the system is under control at a mean fill rate of \(\mu=\mathrm{E}(\mathrm{Z})=16.03\) ounces and a standard deviation of \(\sigma=.01\), so that it is highly improbable that a package is filled below its stated contents of 16 ounces. They want you to test the hypothesis that the filling system is under control at a level of significance equal to .10. They provide you with the following random sample outcome of 40 observations on fill weights:

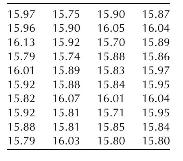
(a) Define a size 10 GLR test of the hypothesis \(H_{0}\) : \(\mu=\mu_{0}, \sigma=\sigma_{0}\) versus \(H_{a}\) : not \(H_{0}\). Test the hypothesis that the filling process is under control. You may use the asymptotic distribution of the GLR if you wish.

(b) Define a size 05 LM test of the same hypothesis as in

(a) above, and test the hypothesis at significance level .10. Are the two tests different? Are the two test decisions in agreement?

(c) Test the two hypotheses \(H_{0}: \mu=16.03\) and \(H_{0}\) : \(\sigma=.01\) individually using size .05 tests. Use whatever test procedures you feel are appropriate. Interpret the outcomes of the tests individually. Interpret the tests jointly using a Bonferroni approach.

(d) Can you define a Wald test for the hypothesis in (a)? If so, perform a Wald test of the joint null hypothesis at significance level . 10 .

Transcribed Image Text:

15.90 15.87 15.90 16.05 16.04 15.70 15.89 15.88 15.86 15.83 15.97 15.84 15.95 16.01 16.04 15.92 15.81 15.71 15.95 15.88 15.81 15.85 15.84 15.79 16.03 15.80 15.80 15.97 15.75 15.96 16.13 15.92 15.79 15.74 16.01 15.89 15.92 15.88 15.82 16.07