Consider a Stirling cycle from Example 8.10 with imperfect regenerator (left(T_{R}=230^{circ} mathrm{C} ight)). Assume ideal gas. a.
Question:
Consider a Stirling cycle from Example 8.10 with imperfect regenerator \(\left(T_{R}=230^{\circ} \mathrm{C}\right)\). Assume ideal gas.
a. Compute the efficiency from
\[ \eta=\frac{\text { Work }_{\text {net }}}{\text { Heat }_{\text {input }}} \]
where
\[ \text { Heat }_{\text {input }}=q_{34}+m C_{v}\left(T_{H}-T_{R}\right) \]
Verify that the efficiency you get is the same as the efficiency obtained by Equation 8.61.
b. Start from the earlier efficiency equation. Derive the efficiency in Equation 8.61.
Example 8.10
A Stirling engine with air as the working fluid operates at a source temperature of 400°C
and a sink temperature of 80°C. The compression ratio is 5.
Assuming perfect regeneration, determine the following:
1. Expansion work.
2. Heat input.
3. Compression work.
4. Efficiency of the machine.
If the regenerator temperature is 230°C, determine
5. The regenerator effectiveness.
6. Efficiency of the machine.
7. If the regeneration effectiveness is zero, what is the efficiency of the machine?
Equation 8.61
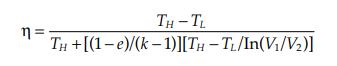
Step by Step Answer:






