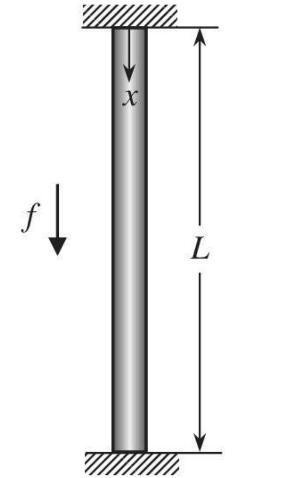
A vertical rod of elastic material is fixed at both ends with constant crosssectional area \(A\), Young's modulus \(E\), and height of \(L\) under the distributed load \(f\) per unit length. The vertical deflection \(u(x)\) of the rod is governed by the following differential equation:

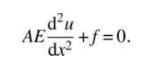
Using three elements of equal length, solve for \(u(x)\) and compare it with the exact solution. Use the following numerical values: \(A=10^{4} \mathrm{~m}^{2}, E=10 \mathrm{GPa}, L=0.3 \mathrm{~m}, f=10^{6} \mathrm{~N} / \mathrm{m}\).

Transcribed Image Text:

du AE +f=0. dr