Solve example 8.5 using Newmark's method with two different time steps (Delta t=42 mu) sec and (Delta
Question:
Solve example 8.5 using Newmark's method with two different time steps \(\Delta t=42 \mu\) sec and \(\Delta t=75 \mu \mathrm{sec}\). Compare the tip displacement using the average acceleration method (unconditionally stable) and the linear acceleration method (conditionally stable).
Data From Exercise 8.5:
Consider a clamped uniaxial bar subjected to a tip load as shown in figure 8.9. Use three elements of equal length to determine the tip displacement as a function of time. Use the central difference method for time integration and the consistent mass matrix. The properties of the bar are: \(E=100 \mathrm{GPa}, L=1 \mathrm{~m}, A=10^{-5} \mathrm{~m}^{2}, ho=4000 \mathrm{~kg} / \mathrm{m}^{3}\). The tip force is given by: \(F(t)=2 F_{\text {max }} t / t_{\max }\) for \(0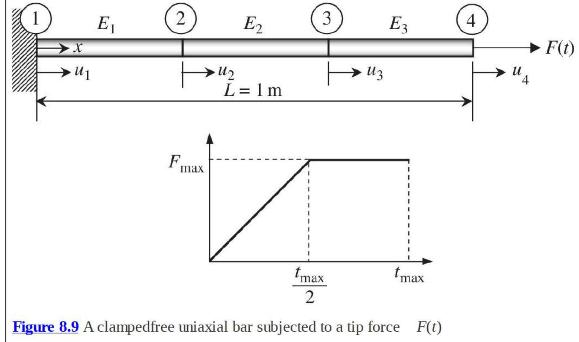
Step by Step Answer:

Introduction To Finite Element Analysis And Design
ISBN: 9781119078722
2nd Edition
Authors: Nam H. Kim, Bhavani V. Sankar, Ashok V. Kumar





