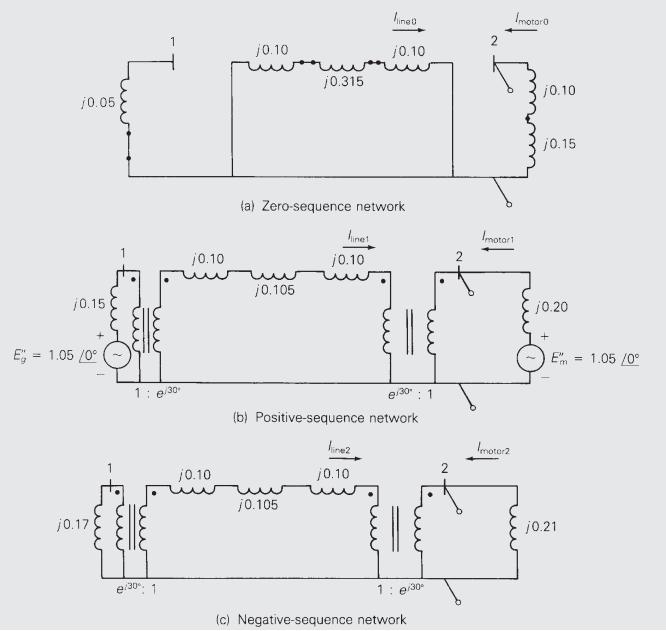
Compute the \(4 \times 4\) per-unit zero-, positive-, and negative-sequence bus impedance matrices for the power system given in Problem 9.5. Use a base of \(1000 \mathrm{MVA}\) and \(20 \mathrm{kV}\) in the zone of generator G3.

Problem 9.5

Equipment ratings for the four-bus power system shown in Figure 7.14 are given as follows:

Generator G1: \(\quad 500 \mathrm{MVA}, 13.8 \mathrm{kV}, \mathrm{X}_{d}^{\prime \prime}=\mathrm{X}_{2}=0.20, \mathrm{X}_{0}=0.10\) per unit 

Generator G2: \(750 \mathrm{MVA}, 18 \mathrm{kV}, \mathrm{X}_{d}^{\prime \prime}=\mathrm{X}_{2}=0.18, \mathrm{X}_{0}=0.09\) per unit 

Generator G3: \(1000 \mathrm{MVA}, 20 \mathrm{kV}, \mathrm{X}_{d}^{\prime \prime}=0.17, \mathrm{X}_{2}=0.20, \mathrm{X}_{0}=0.09\) per unit 

Transformer T1: \(500 \mathrm{MVA}, 13.8 \mathrm{kV} \Delta / 500 \mathrm{kV} \mathrm{Y}, \mathrm{X}=0.12\) per unit 

Transformer T2: \(750 \mathrm{MVA}, 18 \mathrm{kV} \Delta / 500 \mathrm{kV} \mathrm{Y}, \mathrm{X}=0.10\) per unit 

Transformer T3: \(1000 \mathrm{MVA}, 20 \mathrm{kV} \Delta / 500 \mathrm{kV} \mathrm{Y}, \mathrm{X}=0.10\) per unit 

Each line: \(\quad \mathrm{X}_{1}=50\) ohms, (\mathrm{X}_{0}=150 \mathrm{ohms}\)

The inductor connected to generator G3 neutral has a reactance of \(0.028 \Omega\). Draw the zero-, positive-, and negative-sequence reactance diagrams using a \(1000 \mathrm{MVA}, 20-\mathrm{kV}\) base in the zone of generator G3. Neglect \(\Delta-Y\) transformer phase shifts.

Figure 7.14

10.05 10.15 E 1.05 /0 0 = j0.17 1: e/30- 30% 10.10 10.10 j0.10 j0.105 10.315 (a) Zero-sequence network j0.105 line! j0.10 hinga j0.10 (b) Positive-sequence network line2 j0.10 ||| : 1: (c) Negative-sequence network 30 2 2 Imotort Imotor2 Imotoro j0.10 j0.15 j0.20 E j0.21 1.05 /0