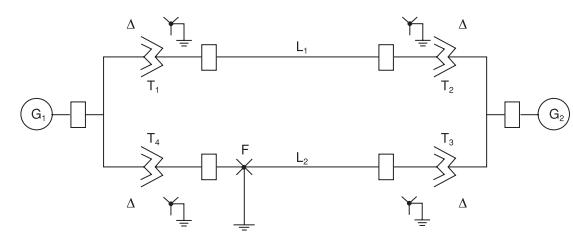
Consider the system shown in Figure 9.18.

(a) As viewed from the fault at \(F\), determine the Thévenin equivalent of each sequence network. Neglect \(\Delta-Y\) phase shifts.

(b) Compute the fault currents for a balanced threephase fault at fault point \(\mathrm{F}\) through three fault impedances \(Z_{\mathrm{FA}}=Z_{\mathrm{FB}}=\)  (Z_{\mathrm{FC}}=j 0.5 \) per unit. Equipment data in per-unit on the same base are given as follows:

Synchronous generators:

G1 \(\mathrm{X}_{1}=0.2\)

\(\mathrm{X}_{2}=0.12\)

\(\mathrm{X}_{0}=0.06\)

G2 \(\quad \mathrm{X}_{1}=0.33\)

\(\mathrm{X}_{2}=0.22\)

\(\mathrm{X}_{0}=0.066\)

Transformers:

T1 \(\quad \mathrm{X}_{1}=\mathrm{X}_{2}=\mathrm{X}_{0}=0.2\)

T2 \(\quad \mathrm{X}_{1}=\mathrm{X}_{2}=\mathrm{X}_{0}=0.225\)

T3 \(\quad \mathrm{X}_{1}=\mathrm{X}_{2}=\mathrm{X}_{0}=0.27\) 

T4 \(\quad \mathrm{X}_{1}=\mathrm{X}_{2}=\mathrm{X}_{0}=0.16\)

Transmission lines:

\(\begin{array}{lll}\text { L1 } & \mathrm{X}_{1}=\mathrm{X}_{2}=0.14 & \mathrm{X}_{0}=0.3 \\ \text { L1 } & \mathrm{X}_{1}=\mathrm{X}_{2}=0.35 & \mathrm{X}_{0}=0.6\end{array}\)

Figure 9.18

Transcribed Image Text:

G F L L T HIG