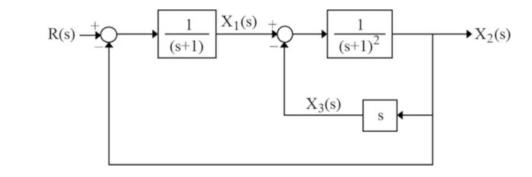
Determine the state equations of the control system shown in Fig. 1.6.

1) \(\frac{d}{d t}\left[\begin{array}{l}x_{1} \\ x_{2} \\ x_{3}\end{array}ight]=\left[\begin{array}{ccc}-1 & -1 & 0 \\ 0 & 0 & 1 \\ 1 & -1 & -3\end{array}ight]\left[\begin{array}{l}x_{1} \\ x_{2} \\ x_{3}\end{array}ight]+\left[\begin{array}{l}1 \\ 0 \\ 0\end{array}ight] u(t)\)
2) \(\frac{d}{d t}\left[\begin{array}{l}x_{1} \\ x_{2} \\ x_{3}\end{array}ight]=\left[\begin{array}{ccc}-1 & -1 & 0 \\ 0 & 0 & 1 \\ 0 & 0 & 1\end{array}ight]\left[\begin{array}{l}x_{1} \\ x_{2} \\ x_{3}\end{array}ight]+\left[\begin{array}{l}1 \\ 0 \\ 0\end{array}ight] u(t)\)
3) \(\frac{d}{d t}\left[\begin{array}{l}x_{1} \\ x_{2} \\ x_{3}\end{array}ight]=\left[\begin{array}{ccc}-1 & -1 & 0 \\ 1 & -1 & 1 \\ 1 & 0 & 1\end{array}ight]\left[\begin{array}{l}x_{1} \\ x_{2} \\ x_{3}\end{array}ight]+\left[\begin{array}{l}1 \\ 0 \\ 0\end{array}ight] u(t)\)
4) \(\frac{d}{d t}\left[\begin{array}{l}x_{1} \\ x_{2} \\ x_{3}\end{array}ight]=\left[\begin{array}{ccc}-1 & -1 & 0 \\ 0 & 0 & 1 \\ 1 & -1 & 2\end{array}ight]\left[\begin{array}{l}x_{1} \\ x_{2} \\ x_{3}\end{array}ight]+\left[\begin{array}{l}1 \\ 0 \\ 0\end{array}ight] u(t)\)

Figure 1.6

Transcribed Image Text:

R(s) (s+1) X1(s) X3(s) (s+1)2 X2(s)