The state equations of a control system are as follows. Determine the state-transition matrix of the system \((\boldsymbol{\varphi}(t))\).

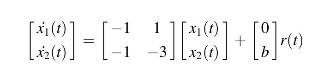
1) \(\left[\begin{array}{cc}(1+t) e^{-2 t} & t e^{-2 t} \\ t e^{-2 t} & (1-t) e^{-2 t}\end{array}ight]\)
2) \(\left[\begin{array}{cc}2 e^{-2 t} & e^{-2 t} \\ -e^{-2 t} & -2 e^{-2 t}\end{array}ight]\)
3) \(\left[\begin{array}{cc}(1+t) e^{-2 t} & t e^{-2 t} \\ -t e^{-2 t} & (1-t) e^{-2 t}\end{array}ight]\)
4) The value of \(b\) is needed to determine the state-transition matrix.

Transcribed Image Text:

[x2(1) 1-3 r(t)