Calculation practice: Confidence interval for variance. Refer to Practice Problem 1. Using the data, calculate the 95% confidence interval for the variance with the following steps.

a. What does the confidence interval refer to—the sample variance or the population variance?

b. What assumptions are necessary to apply the formula discussed in this chapter for the confidence interval for variance?

c. What is the sample variance of these data?

d. How many degrees of freedom are associated with these data for estimates of variance?

e. What is α^α for this analysis?

f. Find the critical value of χ² for α/2.

g. What is the critical value of χ² for 1−α/2?

h. Calculate the 95% confidence interval for the variance of the elevational range shift.

Data from problem 1

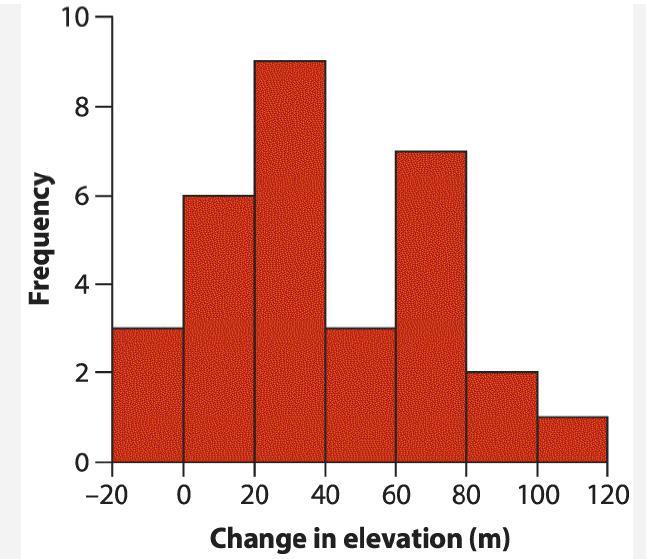
Calculation practice: Confidence interval for a mean and one-sample t-test As the world warms, the geographic ranges of species might shift toward cooler areas. Chen et al. (2011), studied recent changes in the highest elevation at which species occur. Typically, higher elevations are cooler than lower elevations. Below are the changes in highest elevation for 31 taxa, in meters, over the late 1900s and early 2000s. (Many taxa were surveyed, including plants, vertebrates, and arthropods.) Positive numbers indicate upward shifts in elevation, and negative numbers indicate shifts to lower elevations. The values are displayed in the accompanying figure.

Transcribed Image Text:

58.9,58.9, 7.8,7.8, 108.6,108.6, 44.8.44.8, 11.1.11.1, 19.2.19.2, 61.9,61.9, 30.530.5 12.7.12.7, 35.8,35.8, 7.4.7.4, 39.3.39.3, 24.0,24.0, 62.1,62.1, 24.3.24.3, 55.3 55.3 32.7,32.7, 65.3,65.3, -19.3.-19.3, 7.6.7.6, -5.2. -2.1.-5.2, -2.1, 31.0,31.0, 69.069.0 88.6,88.6, 39.5,39.5, 20.7,20.7, 89.0,89.0, 69.0,69.0, 64.9,64.9, 64.864.8