Consider the familiar double-slit experiment: a quantum mechanical particle of mass \(m\) starts at some position to the left of the slits at time \(t=0\). As time evolves, the wavefunction of the particle passes through the slits and continues to a screen at which the particle is observed. This configuration is illustrated in Fig. 11.7. At \(t=0\), the particle is located a distance \(d\) to the left of the slits,

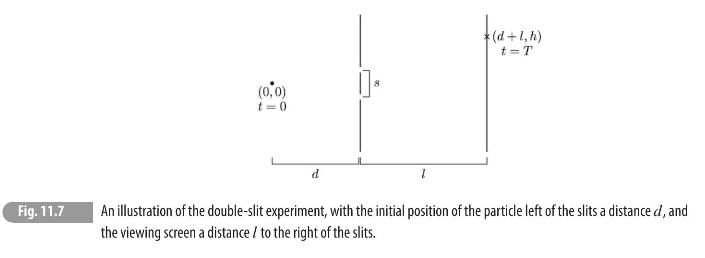
directly in between them vertically. The slits are separated by a distance \(s\). The screen is located a distance \(l\) to the right of the slits, and the particle is observed at time \(t=T\) at a position \((d+l, h)\) with respect to the initial point. From the initial to final point the particle travels freely, under the influence of no potential.
(a) First, calculate the two path integrals corresponding to the two possible paths to the screen: through the upper or lower slit. Note that this is a two-dimensional problem.
(b) We do not measure which slit the particle passes through, so we need to sum these two path integrals as probability amplitudes. Do this, and determine the probability distribution for the particle to be detected at a location on the screen. Does this result seem familiar? Sketch a plot of the distribution you find as a function of the distance away from the center of the screen, \(h\).

Transcribed Image Text:

Fig. 11.7 8 (0,0) t=0 d (d+l,h) t-T An illustration of the double-slit experiment, with the initial position of the particle left of the slits a distanced, and the viewing screen a distance / to the right of the slits.