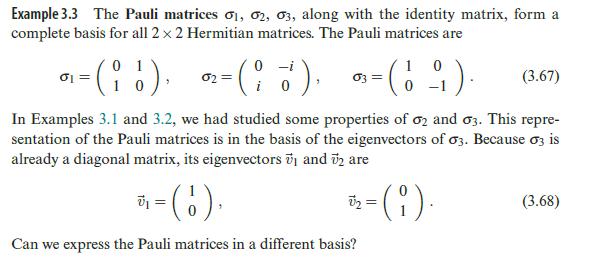
In this exercise, we study the unitary matrix constructed from exponentiation of one of the Pauli matrices, introduced in Example 3.3. As Hermitian matrices, exponentiation should produce a unitary matrix, and we will verify that here.

(a) As Hermitian matrices, the Pauli matrices can be exponentiated to construct a corresponding unitary matrix. Let's exponentiate \(\sigma_{3}\) as defined in Eq. (3.67) to construct the matrix

\[\begin{equation*}\mathbb{A}=e^{i \phi \sigma_{3}} \tag{3.150}\end{equation*}\]

where \(\phi\) is a real number. What is the resulting matrix \(\mathbb{A}\) ? Write it in standard \(2 \times 2\) form. Is it actually unitary? Remember that exponentiation of a matrix is defined by its Taylor expansion.

(b) Now, consider the Hermitian matrix constructed from the sum of \(\sigma_{1}\) and \(\sigma_{3}:\)
\[\sigma_{1}+\sigma_{3}=\left(\begin{array}{cc}1 & 1 \tag{3.151}\\1 & -1\end{array}\right)\]
Exponentiate this matrix to construct \(\mathbb{B}\), where \[\begin{equation*}\mathbb{B}=e^{i \phi\left(\sigma_{1}+\sigma_{3}\right)} \tag{3.152}\end{equation*}\]
Can you express the result in the form of a \(2 \times 2\) matrix?
Taylor expand the exponential function and sum up the terms with even powers of \(\phi\) and odd powers of \(\phi\) separately.

Example 3.3.

Example 3.3 The Pauli matrices 61, 62, 63, along with the identity matrix, form a complete basis for all 22 Hermitian matrices. The Pauli matrices are 0 1 0-i i 0 1 0 = ( ) = ( )=(69). 0 02= (3.67) In Examples 3.1 and 3.2, we had studied some properties of 2 and 03. This repre- sentation of the Pauli matrices is in the basis of the eigenvectors of 3. Because 3 is already a diagonal matrix, its eigenvectors 1 and 2 are -(d). Can we express the Pauli matrices in a different basis? 2= = (i). (3.68)