29. Stratified Sampling: Let U1, . . . ,Un be independent random numbers and set U i...
Question:
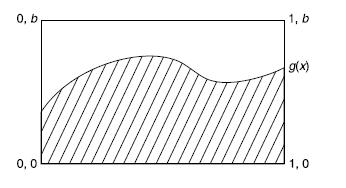
29. Stratified Sampling: Let U1, . . . ,Un be independent random numbers and set
¯U i = (Ui + i − 1), i=1, . . . , n. Hence, ¯Ui , i1, is uniform on ((i − 1), i). ni
=1 g(
¯U i) is called the stratified sampling estimator of
1 0 g(x) dx.
(a) Show that E[ni
=1 g(
¯U i)] =
1 0 g(x) dx.
(b) Show that Var[ni
=1 g(
¯U i)] Var[ni
=1 g(Ui)].
Hint: Let U be uniform (0, 1) and define N by N = i if (i − 1)
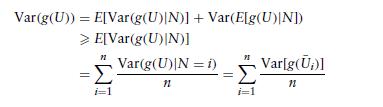
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Related Book For 

Question Posted:






