Let D denote the time between successive departures in a stationary M/M/1 queue with Hint: By

Let D denote the time between successive departures in a stationary M/M/1 queue with λ

Hint: By conditioning on whether or not the departure has left the system empty we see that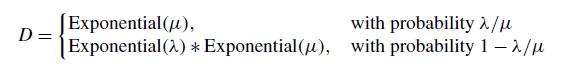

where Exponential(λ) ∗ Exponential(μ) represents the sum of two independent exponential random variables having rates μ and λ. Now use momentgenerating functions to show that D has the required distribution.
Note that the preceding does not prove that the departure process is Poisson.
To prove this we need show not only that the interdeparture times are all exponential with rate λ, but also that they are independent.

Transcribed Image Text:

D = Exponential(), * with probability / Exponential (2) Exponential(u), with probability 1 -/