The chemical potential was defined by (5) as (F/N) a,V . an equivalent expression listed in table 5.1 is = (U/N) a,V Prove that

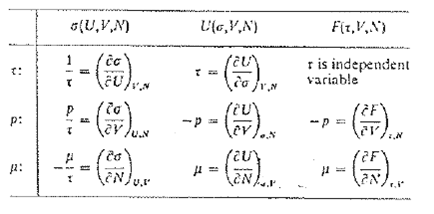
The chemical potential was defined by (5) as (∂F/∂N)_a,V. an equivalent expression listed in table 5.1 is

μ = (∂U/∂N)_a,V

Prove that this relation, which was used by Gibbs to define μ, is equivalent to the definition (5) that we have adopted. It will be convenient to make use of the results (31) and (35). Our reasons for treating (5) as the definition of μ, and (85) as a mathematical consequence, are two-fold. In practice, we need the chemical potential more often as a function of the temperature τ than as a function of the entropy σ. Operationally a process in which a particle is added to a system while the temperature of the system is kept constant is a more natural process than one in which the entropy is kept constant; Adding a particle to a system at a finite temperature tends to increase its entropy unless we can keep each system of the ensemble in a definite, although new, quantum state. There is no natural laboratory process by which this can be done. Hence the definition (5) or (6), in which the chemical potential is expressed as the change in free energy per added particle under conditions of constant temperature is operationally the simpler. We point out that (85) will not give U = μN on integration, because μ(N, σ, V) is a function of N; compare with (9.I3)

3(U,V,N) L'(6,V,N') F(z,V,N) - (). - -- ( ---). r is independent variable t: p: , ,.(N