Two-phase interfacial boundary conditions, in S2.1, boundary conditions for solving viscous flow problems were given. At that
Question:
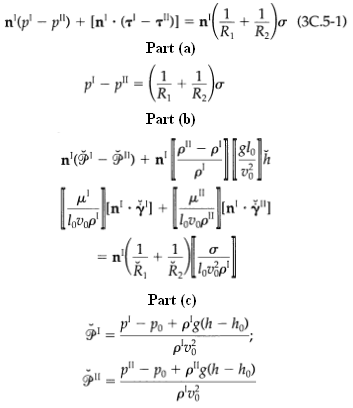
Two-phase interfacial boundary conditions, in S2.1, boundary conditions for solving viscous flow problems were given. At that point no mention was made of the role of interfacial tension. At the interface between two immiscible fluids, I and II, the following boundary condition should be used: This is essentially a momentum balance written for an interfacial element dS with no matter passing through it, and with no interfacial mass or viscosity. Here n1 is the unit vector normal to dS and pointing into phase I. The quantities R1 and R2 are the principal radii of curvature at dS, and each of these is positive if its center lies in phase I. The sum (1/R1) + (1/R2) can also be expressed as (∆ ∙ n1). The quantity σ is the interfacial tension, assumed constant.
(a) Show that, for a spherical droplet of 1 at rest in a second medium II, Laplace's equation relates the pressures inside and outside the droplet, is the pressure in phase I greater than that in phase II, or the reverse? What is the relation between the pressures at a planar interface?
(b) Show that Eq. 3C.5-1 leads to the following dimensionless boundary condition in which h = (h – h0)/l0 is the dimensionless elevation of dS, γ and γ are dimensionless rate-of-deformation tensors, and R1 = R1/l0 and/R2 = R2/l0 are dimensionless radii of curvature. Furthermore
In the above, the zero-subscripted quantities are the scale factors, valid in both phases. Identify the dimensionless groups that appear in Eq. 3C.5-3.
Step by Step Answer:
Twophase interfacial boundary conditions a This result follows at once from ...View the full answer
