A uniformly magnetized and conducting sphere of radius R and total magnetic moment m = 4?MR³/3 rotates about its magnetization axis with angular speed ?. In the steady state no current flows in the conductor. The motion is non-relativistic; the sphere has no excess charge on it.

(a) By considering Ohm's law in the moving conductor, show that the motion induces an electric field and a uniform volume charge density in the conductor, ? = -m?/?c²R³.

(b) Because the sphere is electrically neutral, there is no monopole electric field outside. Use symmetry arguments to show that the lowest possible electric multipolarity is quadrupole. Show that only a quadrupole field exists outside and that the quadrupole moment tensor has nonvanishing components, Q₃₃ = -4m?R²/3c², Q₁₁ = Q₂₂ = -Q₃₃/2.

(c) By considering the radial electric fields inside and outside the sphere, show that the necessary surface-charge density ?(?) is

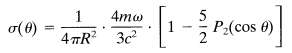
(d) The rotating sphere serves as a unipolar induction device if a stationary circuit is attached by a slip ring to the pole and a sliding contact to the equator. Show that the line integral of the electric field from the equator contact to the pole contact (by any path) is ? = ?₀m?/4?R.

Transcribed Image Text:

4то 1 - P2(cos 6) o(0) 4TR? 3c