For the normal distribution 2k = 2k (2k)! / (k! 2k) and 2k+1 = 0, k =
Question:
For the normal distribution μ2k = σ2k (2k)! / (k! 2k) and μ2k+1 = 0, k = 0, 1, . . . . Use this result to analyze the two estimators where mk = 1/n Σni=1 (xi ?? x)k. The following result will be useful. Asy Cov [??nmj, ??nmk] = μj + k ?? μjμk + jkμ2μj??1 μk??1 ?? jμj??1μk+1 ?? kμk??1μj+1. Use the delta method to obtain the asymptotic variances and covariance of these two functions assuming the data are drawn from a normal distribution with mean μ and variance σ2. Under the assumptions, the sample mean is a consistent estimator ofμ, so for purposes of deriving asymptotic results, the difference between x and μ may be ignored. As such, no generality is lost by assuming the mean is zero, and proceeding from there. Obtain V, the 3 × 3 covariance matrix for the three moments, then use the delta method to show that the covariance matrix for the two estimators is where J is the 2 x 3 matrix of derivatives.
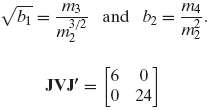
The word "distribution" has several meanings in the financial world, most of them pertaining to the payment of assets from a fund, account, or individual security to an investor or beneficiary. Retirement account distributions are among the most...
Step by Step Answer:






