Force on a surface of arbitrary orientation? (Figure 1D.2) Consider the material within an element of volume

Question:

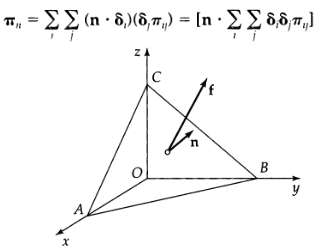
Force on a surface of arbitrary orientation? (Figure 1D.2) Consider the material within an element of volume OABC that is in a state of equilibrium, so that the sum of the forces acting on the triangular faces ?OBC, ?OCA, ?OAB, and ?ABC must be zero. Let the area of ?ABC be dS, and the force per unit area acting from the minus to the plus side of dS be the vector ?n. Show that ?n = [n ? ?].

(a) Show that the area of ?OBC is the same as the area of the projection ?ABC on the yz-plane; this is (n ? ?x) dS write similar expressions for the areas of ?OCA and ?OAB.

(b) Show that according to Table 1.2-1 the force per unit area on ?OBC is ?x ?x?, + ?y ?xy + ?z ?xz. Write similar force expressions for ?OCA and ?OAB.

(c) Show that the force balance for the volume element OABC gives in which the indices i, j take on the values x, y, z. The double sum in the last expression is the stress tensor ? written as a sum of products of unit dyads and components. Fig. 1D.2 Element of volume OABC over which a force balance is made. The vector ?n = [n ? ?] is the force per unit area exerted by the minus material (material inside OABC) on the plus material (material outside OABC). The vector n is the outwardly directed unit normal vector on face ABC.