If X 1 is the mean of a random sample of size n from a normal population
Question:
If X̅1 is the mean of a random sample of size n from a normal population with the mean µ and the variance σ21, X̅2 is the mean of a random sample of size n from a normal population with the mean µ and the variance σ22, and the two samples are independent, show that
(a) ω ∙ X̅1 +(1 – ω) ∙ X̅2, where 0 ≤ ω ≤ 1, is an unbiased estimator of µ;
(b) The variance of this estimator is a minimum when
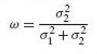
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Answer rating: 80% (21 reviews)
a E w x 1 1 w x 2 ...View the full answer

Answered By

Daniel Kimutai
I am a competent academic expert who delivers excellent writing content from various subjects that pertain to academics. It includes Electronics engineering, History, Economics, Government, Management, IT, Religion, English, Psychology, Sociology, among others. By using Grammarly and Turnitin tools, I make sure that the writing content is original and delivered in time. For seven years, I have worked as a freelance writer, and many scholars have achieved their career dreams through my assistance.
5.00+
1+ Reviews
10+ Question Solved
Related Book For 

John E Freunds Mathematical Statistics With Applications
ISBN: 9780134995373
8th Edition
Authors: Irwin Miller, Marylees Miller
Question Posted:
Students also viewed these Statistics questions
-
Consider the probability statement Where X-bar is the mean of a random sample of size n from N(, 2) distribution with known 2. (a) Find k. (b) Use this statement to find a confidence interval for ....
-
With reference to Exercise 10.21, find the efficiency of the estimator of part (a) with Ï = 1/2 relative to this estimator with In exercise If 1 is the mean of a random sample of size n from a...
-
Show that the mean of a random sample of size n is a minimum variance unbiased estimator of the parameter of a Poisson population.
-
15. The temperature C, in degrees, that is equivalent to a temperature of F degrees Fahrenheit is given by C- graph of this equation shows the temperature in Celsius for the corresponding...
-
a. The variables in Exercise 5.3 are either discrete or continuous. Which are they and why? b. Explain why the variable number of dinner guests for Thanksgiving dinner is discrete. c. Explain why the...
-
please help (a) Vedika Ltd. issued 80,000 8% convertible debentures of 100 each on 14 April, 2015. The debentures are due for redemption on 31st March, 2019 at a premium of 20%, convertible into...
-
Kim Ries, Tere Bax, and Josh Thomas invested $80,000, $112,000, and $128,000, respectively, in a partnership. During its first calendar-year, the firm earned $249,000. Required Prepare the entry to...
-
A portfolio consists of the following four stocks. What is the expected return of theportfolio? Current Expected Return Stock Market Value s 180,000 145,000 452,000 223,000 $1,000,000 8% 10 12
-
View Policies Current Attempt in Progress The Cullumber Acres Inn is trying to determine its break-even point during its off-peak season. The inn has 50 rooms that it rents at $65 a night. Operating...
-
We say that OSPF is a hierarchical intradomain protocol, but RIP is not. What is the reason behind this statement?
-
Rework Example 10.5 using the alternative formula for the information given in Exercise 10.19. Example 10.5 Show that is a minimum variance unbiased estimator of the mean of a normal population.
-
If X 1 and X 2 are the means of independent random samples of sizes n 1 and n 2 from a normal population with the mean and the variance 2 , show that the variance of the unbiased estimator Is a...
-
Dan Lanier is a certified public accountant (CPA) and staff accountant for Baker and Lin, a local CPA firm. It had been the policy of the firm to provide a holiday bonus equal to two weeks' salary to...
-
(ii) State Wilkie's updating equation in respect of the force of inflation and explain carefully what each of the components of the equation represents. State also which type of time series process...
-
Compute the double integral D x y dA over the domain D indicated as 0 x 5, x y 2x + 3. (Use symbolic notation and fractions where needed.) f(x, y) A = D
-
4. (10 points) A researcher believes that length of time spent listening to classical music increases memory for previously learned material. She has 4 groups of 5 subjects listen to either 10 min.,...
-
We find a binary system consisting of a 1 solar mass star, still in its main sequence phase, and a white dwarf. Assume both stars formed at the same time and that they did not significantly influence...
-
The equity sections from Atticus Group's 2015 and 2016 year-end balance sheets follow. Stockholders Equity (December 31, 2015) Common stock $6 par value, 50,000 shares authorized, 35,000 shares...
-
Go to www.brighthubpm.com/monitoring-projects/51982-understanding-the-s-curve-theory-for-project-management-monitoring/ and read the article on the multiple uses of project S-curves. What does the...
-
A police officer pulls you over and asks to search your vehicle because he suspects you have illegal drugs inside your car. Since he doesn't have reasonable suspicion to search your car, legally he...
-
GM Financial, General Motors Company's financing segment, has special purpose entities that are consolidated in GM's financial statements. GM Financial transfers receivables and lease-related assets...
-
The following contingency table summarizes the number of students at a college who have a Mastercard or a Visa credit card. a. Find the probability that a randomly selected student ( 1) Has a...
-
The card game of Euchre employs a deck that consists of all four of each of the aces, kings, queens, jacks, tens, and nines (one of each suit clubs, diamonds, spades, and hearts). Find the...
-
Each month a brokerage house studies various companies and rates each companys stock as being either low risk or moderate to high risk. In a recent report, the brokerage house summarized its findings...
-
QUESTION 3 A business owns seven flats rented out to staff at R500 per month. All flats were tenanted Ist january 21 months rent was in arrears and as at 31st December 14 months' rent wa Identify the...
-
1. 2. 3. Select the Tables sheet, select cells A6:B10, and create range names using the Create from Selection button [Formulas tab, Defined Names group]. Select cells B1:F2 and click the Name box....
-
Tropical Rainwear issues 3,000 shares of its $18 par value preferred stock for cash at $20 per share. Record the issuance of the preferred shares. (If no entry is required for a particular...

Study smarter with the SolutionInn App


