In three dimensions the solution to the wave equation (6.32) for a point source in space and time (a light flash at t' = 0, x' = 0) is a spherical shell disturbance of radius R = ct, namely the Green function G⁽⁺⁾ (6.44). It may be initially surprising that in one or two dimensions, the disturbance possesses a "wake," even though the source is a "point" in space and time. The solutions for fewer dimensions than three can be found by superposition in the superfluous dimension(s), to eliminate dependence on such variable(s). For example, a flashing line source of uniform amplitude is equivalent to a point source in two dimensions.

(a) Starting with the retarded solution to the three-dimensional wave equation (6.47), show that the source f(x?, t') = ?(x')?(y')?(f), equivalent to a t = 0 point source at the origin in two spatial dimensions, produces a two-dimensional wave,

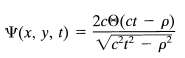
where ?² = x² + y² and ?(?) is the unit step function [?(?) = 0 (1) if ? )0.]

(b) Show that a "sheet" source, equivalent to a point pulsed source at the origin in one space dimension, produces a one-dimensional wave proportional to

?(?, t) = 2?c?(ct - |x|)

- p) 2c0(ct Ver - p Y(x, y, t) =