Let the r v s Xj, j yen 1, be distributed as follows Show that the Lindeberg condition (relation (12 24)) holds, if and only if Icirc plusmn 3 2 Conclude that For Icirc plusmn 3 2, show that which is implied by n 2a Eacute 2 s n 2 for large n, so that g n ( Eacute ) 0 Next, g n ( Eacute ) yen 1 Eac...

Here X j 0 2 j 2 X j X 2 j 2j 2 6j 21 13 j 2 so that s 2 n nn12n118 Consider js with j 1 ...

Let the r.v.s Xj, j ¥ 1, be distributed as follows: Show that the Lindeberg condition (relation

Question:

Let the r.v.s Xj, j ‰¥ 1, be distributed as follows:

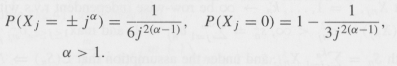
1 1 P(X; = ± jª) = a > 1. P(X; =0) = 1 – 6j2(a-1)' %3D 3 j2(a-1) '

Show that the Lindeberg condition (relation (12.24)) holds, if and only if Î± < 3/2. Conclude that

For Î± < 3/2, show that

which is implied by n^2a < É›²s_n² for large n, so that g_n (É›) = 0. Next,

g_n(É›) ‰¥ 1 - É›²/18 (l €“ 1/k)(2- 1/k) k^2a/É›²s_n² k^3-2a,

Where k = [(É›s_n)^l/Î±], and conclude that the expression on the right-hand side does not converge to 0 for Î± ‰¥ 3/2.

Fantastic news! We've Found the answer you've been seeking!

Step by Step Answer:

Related Book For book-img-for-question

An Introduction to Measure Theoretic Probability

ISBN: 978-0128000427

2nd edition

Authors: George G. Roussas

See More Books

Question Posted: Mar 25, 2016 03:37 AM

See More Questions

Let X n , n ¥ 1, be independent r.v.s distributed as B (1, Ï ), and set Then show that And n- , . X,
For j = 1,2 n, let Xj be independent r.v.s defined as follows: Then show that the Lindeberg condition (condition (12.24)) does not hold. Recall that and show that, for every É > 0 and large n:...
Let Verify that for n ¥ 1, Can either inequality be used to show that S diverges? Show that and conclude that S diverges. n2n
Find the slopes of the curves at the given points. Sketch the curves along with their tangents at these points. r = sin 2; = /4, 3/4
What type of opinion(s) would the audit team issue on the effectiveness of internal control over financial reporting if a material weakness in internal control exists? How would the standard report...
S plc produces and sells three products, X, Y and Z. It has contracts to supply products X and Y, which will utilise all of the specific materials that are available to make these two products during...
A paired difference experiment produced the following results: nd = 38 x1 = 92 x2 =
The income statement of Hauser Company is presented on the shown below.? Additional information: 1. Accounts receivable decreased $290,000 during the year, and inventory increased $140,000. 2....
SECTION A [100 MARKS] Answer ANY FIVE (5) questions in this section. QUESTION 1 Answer the questions from the information provided. Use the information provided below to complete the table for each...
(i) First use the data set 401KSUBS.RAW, keeping only observations with fsize = 1. Find the skewness measure for inc. Do the same for log(mc). Which variable has more skewness and therefore seems...
If the independent r.v.s Xj, j 2, are distributed as follows: P(Xj = -ja) = P(Xj= ja) = 1/j P(Xj=0) = 1 - 2/j (, > 0), Show that the restriction < 1 ensures that the Lindeberg condition (relation...
Let X n , n ¥ 1, be independent r.v.s such that |X n | ¤ M n a.s. with M n = o(s n ) where Set and snow that Hint: From the assumption M n = o(s n ) it follows that so that Mn <...
Civil Code 1719, subdivision (a) provides in part that any person who draws a check that is dishonored due to insufficient funds shall be liable to the payee for the amount owing upon the check and...
Working in the production area of a manufacturing company is right where William feels at home. Several of his family members had worked in similar environments throughout their careers, and he loves...
Use the Nernst equation and reduction potential data from (Petrucci's Appendix D; OpenStax's Table 16.1) to calculate Ecell for the following cell: Al (s) | Al3+ (0.18 M) || Fe+ (0.85 M) | Fe (s)
1.) Describe economic interventions that the federal government used to deal with the COVID shutdown and subsequent inflation. Would you have done anything differently? 2.) Describe three of the...
The goal is to understand the principles of project valuation and capital budgeting in a practical setting, using a combination of fictitious data and real-world examples. Begin by proposing a...
45. The use of realistic predetermined unit costs to facilitate product costing, cost control, cost flow, and inventory valuation is a description of the A. flexible budget concept. B. budgetary...
A speed control system of an engine is shown in Fig. P1.2. Determine: (a) Sensitivity of closed loop system to changes in engine gain $k_{1}$ and tachometer feedback gain $k_{2}$. (b) Steady...
The time to assemble the first unit on a production line is 10 hours. The learning rate is 0.94. Approximately how long will it take for the seventh unit to be assembled? The number of hours needed...
Merck & Co. conducted a study to test the promise of its experimental AIDS vaccine ( The Boston Globe , September 22, 2007). Volunteers in the study were all free of the human immunodeficiency virus...
More and more households are struggling to pay utility bills given a shaky economy and high heating costs (The Wall Street Journal, February 14, 2008). Particularly hard hit are households with homes...
The research team at a leading perfume company is trying to test the market for its newly introduced perfume. In particular the team wishes to look for gender and international differences in the...
Jen bought 100 shares of ABC stock at $15 a share on July 14, 2017. On August 7, 2018, she noticed that the stock had increased in value to $20 a share and decided to sell her shares. Jen's marginal...
Alex. Inci, buys 40 petcent of Steinbart Company on January 1, 2020, for $1.212.000. The equity method of accounting is to be used. Steinbart's net assets on that datewere $2.90 million. Any excess...
exercise 4-7 (Algo) Effects of transactions on income statement LO P2

Let the r.v.s Xj, j ¥ 1, be distributed as follows: Show that the Lindeberg condition (relation

Question:

1 1 P(X; = ± jª) = a > 1. P(X; =0) = 1 – 6j2(a-1)' %3D 3 j2(a-1) '

Step by Step Answer:

Here X j 0 2 j 2 X j X 2 j 2j 2 6j 21 13 j 2 so that s 2 n nn12n118 Consider js with j 1 ...View the full answer

An Introduction to Measure Theoretic Probability

Students also viewed these Statistics questions