A permutation matrix is one that can be obtained from an identity matrix by reordering its rows.
Question:
A permutation matrix is one that can be obtained from an identity matrix by reordering its rows. If P is an n x n permutation matrix and A is any n x n matrix and C = PA, then C can be obtained from A by making precisely the same reordering of the rows of A as the reordering of the rows which produced P from In.
a. Show that every finite group of order n is isomorphic to a group consisting of n x n permutation matrices under matrix multiplication.
b. For each of the four elements e, a, b, and c in the Table 5.11 for the group V, give a specific 4 x 4 matrix that corresponds to it under such an isomorphism.
Table 5.11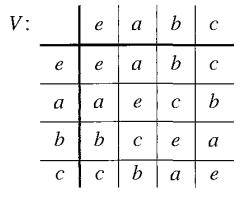
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Related Book For 

Question Posted:





