Compute 2 ( 2 (P 1 P 2 P 3 P 4 )) and show that
Question:
Compute ∂2(∂2(P1P2P3P4)) and show that it is 0, completing the proof of Theorem 41.9.
Data from Theorem 41.9
Let X be a simplicial complex, and let Cn(X) be then-chains of X for n = 0, 1, 2, 3. Then the composite homomorphism ∂n-1∂n mapping Cn(X) into Cn-2(X) maps everything into 0 for n = 1, 2, 3. That is, for each c ∈ Cn(X) we have ∂n-1 (∂n(c)) = 0. We use the notation "∂n-1∂n= 0," or, more briefly, "∂2 = 0."
Proof Since a homomorphism is completely determined by its values on generators, it is enough to check that for an n-simplex σ, we have ∂n-1 (∂n(σ)) = 0. For n = 1 this is obvious, since ∂0 maps everything into 0. For n = 2,
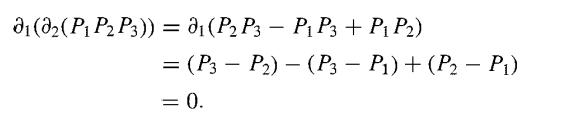
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Related Book For 

Question Posted:





