Following the ideas of Exercises 10, 11, and 12, define the n-dimensional cohomology group H (n) (X)
Question:
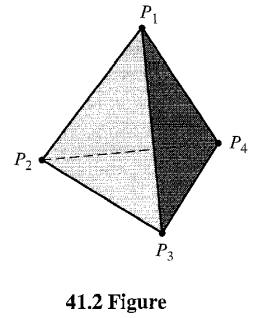
Following the ideas of Exercises 10, 11, and 12, define the n-dimensional cohomology group H(n)(X) of X. Compute H(n)(S) for the surface S of the tetrahedron of Fig. 41.2.
Data from Exercise 10
Let X be a simplicial complex. For an (oriented) n-simplex σ of X, the coboundary δn(σ) of σ is the (n + 1) chain ∑τ, where the sum is taken over all (n + 1)-simplexes τ that have σ as a face. That is, the simplexes τ appearing in the sum are precisely those that have σ as a summand of ∂n+ 1 (τ). Orientation is important here. Thus P2 is a face of P1P2, but P1 is not. However, P1 is a face of P2P1. Let X be the simplicial complex consisting of the solid tetrahedron of Fig. 41.2.
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Related Book For 

Question Posted:





