Assume the hypothesis of Theorem 1 and assume that y₁(x) and y₂(x) are both solutions to the linear first-order equation satisfying the initial condition y(x₀) = y₀.

a. Verify that y(x) = y₁(x)– y₂(x) satisfies the initial value problem:

b. For the integrating factor μ(x) defined by Equation (11.63), show that:

Equation 11.63

c. From part (a), we have y₁(x₀)–y₂(x₀) =0. Since μ(x) > 0 forα 1(x_{) y2}(x)≡ 0 on the interval; (α,β) Thus y₁(x) = y₂(x) for allα

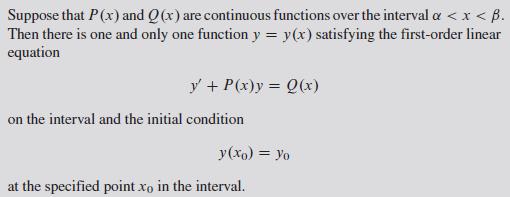
Data from theorem 1

Transcribed Image Text:

y' + P(x) y = 0, y(x) = 0