Assume we have a lake that is stocked with both bass and trout. Because both eat the same food sources, they are competing for survival. Let B(t) and T (t) denote the bass and trout populations, respectively, at time t. The rates of growth for bass and for trout are estimated by the differential equations:

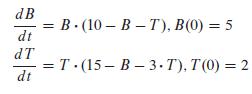
Use Euler's method with step size Δt = 0.1 to estimate the solution curves from 0≤ t ≤7 for

a. B(t) versus t.

b. T (t) versus t.

c. The solution trajectory (B(t), T (t)) in the phase plane.

Transcribed Image Text:

dB dt dT dt = B. (10-B-T), B(0) = 5 = T (15-B-3.T), 7(0) = 2