For Example, 2, show that the optimal value of c is c^∗= 8/ 9. Apply the definition of the absolute value to obtain a piecewise continuous function. Then find the minimum value of the function over the interval [0, 3].

Data from example 2

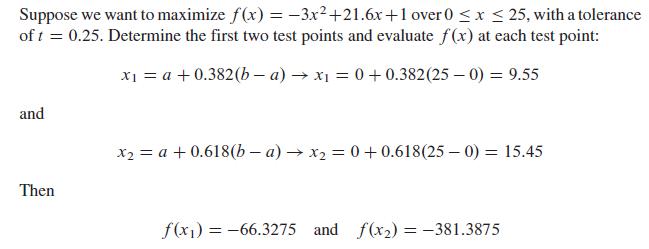
Because f (x₁) > f (x₂), we discard all values in [x₂, b] and select the new interval Œ[a, b]= [0, 15.45]. Then x₂ = 9.55, which is the previous x₁, and f (x₂) = –66.2972. We must now find the position of the new test point x₁ and evaluate f (x₁):

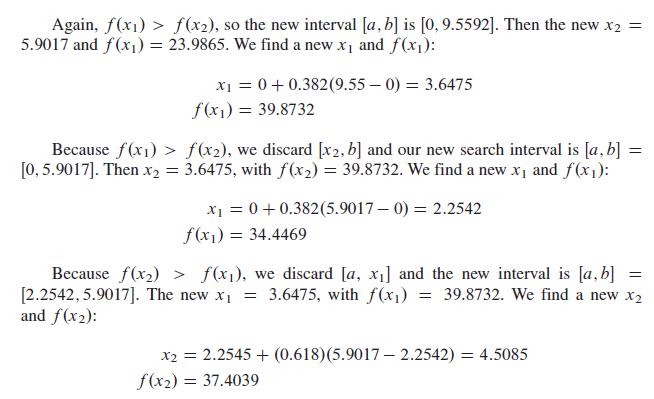
This process continues until the length of the interval of uncertainty, b – a, is less than the tolerance, t = 0.25. This requires 10 iterations. The results of the Golden Section Search Method for Example 2 are summarized in Table 7.4.

Table 7.4

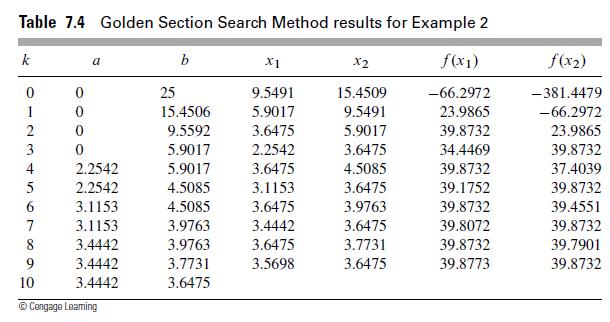 The final interval [a, b] = [3.4442, 3.6475 is the first interval of our [a, b] intervals that is less than our 0.25 tolerance. The value of x that maximizes the given function over the interval must lie within this final interval of uncertainty [3.4442, 3.6475]. We estimate x^∗= (3.4442 + 3.6475)/2 = 3. 5459 and f (x^∗) = 39.8712. The actual maximum, which in this case can be found by calculus, occurs at x^∗   = 3.60, where f (3.60) = 39.88.

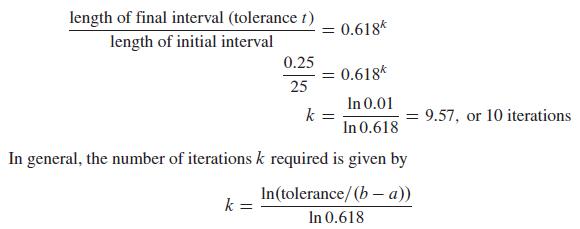
As illustrated, we stopped when the interval of uncertainty was less than 0.25. Alternatively, we can compute the number of iterations required to attain the accuracy specified by the tolerance. Because the interval of uncertainty is 61.8% of the length of the interval
of uncertainty at each stage, we have: