In 1868, the accidental introduction into the United States of the cottony-cushion insect (Icerya purchasi) from Australia threatened to destroy the American citrus industry.
To counteract this situation, a natural Australian predator, a ladybird beetle (Novius cardinalis), was imported. The beetles kept the insects to a relatively low level. When DDT (an insecticide) was discovered to kill scale insects, farmers applied it in the hope of reducing the scale insect population even further. However, DDT turned out to be fatal to the beetle as well, and the overall effect of using the insecticide was to increase the numbers of the scale insect. Let Cn and Bn represent the cottony-cushion insect and ladybird beetle population levels, respectively, after n days. Generalizing the model in Problem 4, we have

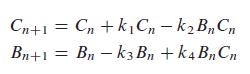
where the k_i are positive constants.

a. Discuss the meaning of each ki in the predator prey model.

b. What assumptions are implicitly being made about the growth of each species in the absence of the other species?

c. Pick values for your coefficients and try several starting values. What is the long-term behavior predicted by your model? Vary the coefficients. Do your experimental results indicate that the model is sensitive to the coefficients? To the starting values?

d. Modify the predator prey model to reflect a predator prey system in which farmers apply (on a regular basis) an insecticide that destroys both the insect predator and the insect prey at a rate proportional to the numbers present.

Data from problem 4

Suppose the spotted owls' primary food source is a single prey: mice. An ecologist wishes to predict the population levels of spotted owls and mice in a wildlife sanctuary. Letting Mn represent the mouse population after n years and O_n the predator owl population, the ecologist has suggested the model.

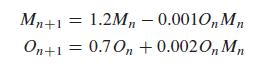
The ecologist wants to know whether the two species can coexist in the habitat and whether the outcome is sensitive to the starting populations. Find the equilibrium values of the dynamical system for this predator-prey model.

Transcribed Image Text:

C+1 Cn + k₁ Cn - K₂ Bn Cn = Bn+1 Bn - k3 Bn + k4BnCn