The operating processes of a spark-ignition engine can be represented by the Otto cycle, which is internally reversible and gives a thermal efficiency of

\[\eta_{\text {Otto }}=1-\frac{1}{r^{(\kappa-1)}}\]

where \(r=\) volumetric compression ratio; \(\kappa=\) ratio of specific heats, \(c_{p} / c_{v}\).

An Otto cycle is depicted in Fig. P6.5, and the temperatures of the two reservoirs associated with the cycle are shown as \(T_{\mathrm{H}}\) and \(T_{\mathrm{C}}\). The thermal efficiency of a Carnot cycle operating between these two reservoirs is \(\eta=1-T_{\mathrm{C}} / T_{\mathrm{H}}\). This value is significantly higher than that of the Otto cycle operating between the same reservoirs. Show the ratio of net work output for the Otto cycle to the energy transferred from the high-temperature reservoir for the Carnot cycle, \(Q_{\mathrm{H}}\), is

\[\eta=\left(1-\frac{1}{r^{(\kappa-1)}}\right) \frac{T_{3}-T_{2}}{T_{3} \ln \left(T_{3} / T_{2}\right)}\]

where \(T_{2}\) and \(T_{3}\) are defined in Fig. P6.5.

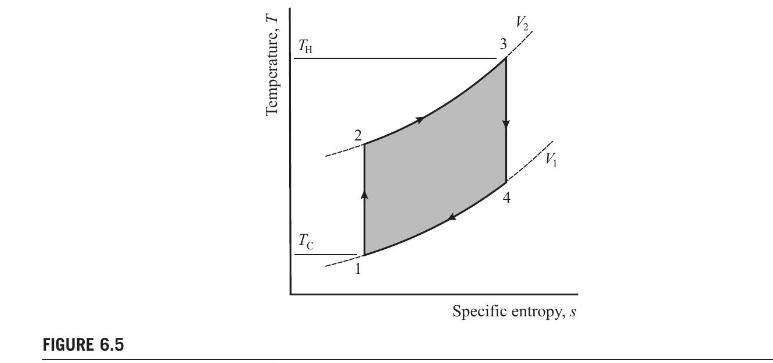
Explain why this value of \(\eta\) differs from that of the Otto cycle, and discuss the significance of the term \(\frac{T_{3}-T_{2}}{T_{3} \ln \left(T_{3} / T_{2}\right)}\). Evaluate the entropy change required at the high-temperature reservoir to supply the Otto cycle in terms of the entropy span, \(s_{3}-s_{2}\), of the Otto cycle.
\[\left[\Delta s_{\mathrm{H}}=c_{v} T_{2}\left(e^{\Delta s / c_{v}}-1\right) / T_{\mathrm{H}}\right]\]

Transcribed Image Text:

FIGURE 6.5 Temperature, T TH N Tc Specific entropy, s