As in Problem 9, consider a planar system of three masses and three identical springs. At equilibrium each mass is at the apex of an equilateral triangle. A spring links each pair of masses.

Two of the masses have mass \(m\) and the third has mass \(2 m\). What are the symmetry operations of the system?

Data from Problem 9

Consider a planar system of three identical masses and three identical springs. At equilibrium each mass is at the apex of an equilateral triangle. A spring links each pair of masses.

Here is its character table (inversions omitted).

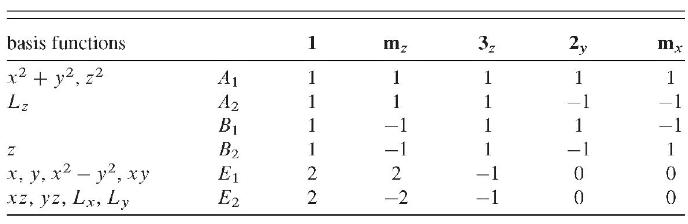
Use coordinate displacements as the basis for a reducible representation. In this representation what are the characters of each of the symmetry operations listed in the table?

Transcribed Image Text:

+4 y