For each of the following subsets S ⊂ R³,

(i) Compute a fairly dense sample of data points z_i ∈ S; 

(ii) Find the principal components of your data set, using μ = .95 in the criterion in (8.78);

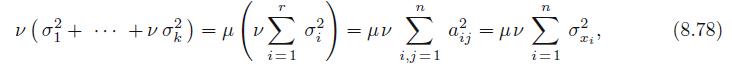
(iii) Using your principal components, estimate the dimension of the set S. Does your estimate coincide with the actual dimension? If not, explain any discrepancies.(a) The line segment S = {(t + 1, 3t − 1,−2t)^T | −1 ≤ t ≤ 1};(b) The set of points z on the three coordinate axes with Euclidean norm ΙΙ z ΙΙ ≤ 1;(c) The set of “probability vectors” S = {(x, y, z)^T | 0 ≤ x, y, z ≤ 1, x+ y + z = 1};(d) The unit ball S = {z ΙΙ ≤ ΙΙ1} for the Euclidean norm;(e) The unit sphere S = {ΙΙ z ΙΙ = 1} for the Euclidean norm;(f) The unit ball S = {ΙΙ z ΙΙ_∞ ≤ 1} for the ∞ norm;(g) The unit sphere S = {ΙΙ z ΙΙ_∞ = 1} for the ∞ norm.

v(0+ +v6) = (2) = ; = - = i=1 i,j=1 n u i=1 19 (8.78)