An article in Health Economics [Estimation of the Transition Matrix of a Discrete-Time Markov Chain (2002, Vol.11,

An article in Health Economics [€œEstimation of the Transition Matrix of a Discrete-Time Markov Chain€ (2002, Vol.11, pp. 33€“42)] considered the changes in CD4 white blood cell counts from one month to the next. The CD4 count is an important clinical measure to determine the severity of HIV infections. The CD4 count was grouped into three distinct categories: 0€“49, 50€“74, and ‰¥ 75. Let X and Y denote the (category minimum) CD4 count at a month and the following month, respectively. The conditional probabilities for Y given values for X were provided by a transition probability matrix shown in the following table.

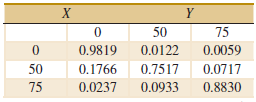
This table is interpreted as follows. For example, P(Y = 50 | X = 75) = 0.0717. Suppose also that the probability distribution for X is P(X = 75) = 0.9,P(X = 50) = 0.08,P(X = 0) = 0.02. Determine the following:

(a) P(Y ‰¤ 50 | X = 50) 

(b) P(X = 0,Y = 75)

(c) E(Y | X = 50) 

(d) f_Y (y) 

(e) f_XY (x, y)

(f) Are X and Y independent?

Transcribed Image Text:

50 75 0.0059 0.9819 0.0122 50 75 0.7517 0.0933 0.1766 0.0237 0.0717 0.8830