In the game of football (“soccer” in the US), a player who is awarded a penalty kick scores about 3/4 of the time. Suppose we model a penalty kick as a game between two players, the shooter, S, and the goalkeeper, G. The shooter has 4 possible actions:

LC: Aim for left corner of the goal.

LM: Aim for left middle of the goal.

RM: Aim for right middle of the goal.

RC: Aim for right corner of the goal.

Aiming for a corner risks missing the net completely, but if the shot is on target it is difficult for the goalkeeper to make a save. Aiming more in the middle gives less risk of missing the net, but more chance for a save. The goalkeeper has 3 possible actions:

L: Lean to the shooter’s left.

M: Stay in the middle.

R: Lean to the shooter’s right.

Leaning to one side as the shooter strikes the ball makes it easier to reach a shot to that side, but harder to reach a shot to the other side. The expected percent of goals scored, is as follows:

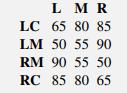
Football is a zero-sum game, so these are the values for S, and the negative of these are the values for G. What are equilibrium strategies for S and G and what is the outcome of the game?

Also, can you come up with a slightly different payoff matrix that results in equilibrium strategies where both players use at least three of their available actions at least some of the time?

Transcribed Image Text:

LMR LC 65 80 85 LM 50 55 90 RM 90 55 50 RC 85 80 65