A researcher measured heart rate \((x)\) and oxygen uptake \((y)\) for one person under varying exercise conditions. He wishes to determine if heart rate, which is easier to measure, can be used to predict oxygen uptake. If so, then the estimated oxygen uptake based on the measured heart rate can be used in place of the measured oxygen uptake for later experiments on the individual:

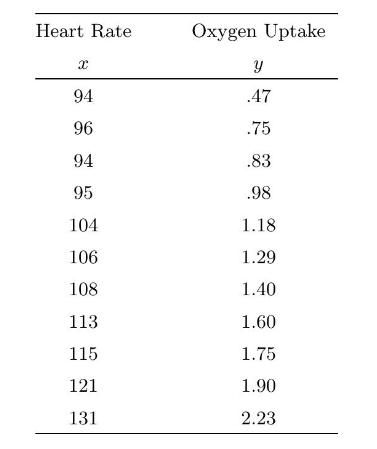
(a) Plot a scatterplot of oxygen uptake \(y\) versus heart rate \(x\).
(b) Calculate the parameters of the least squares line.
(c) Graph the least squares line on your scatterplot.
(d) Calculate the estimated variance about the least squares line.
(e) Suppose that we know that oxygen uptake given the heart rate is emphnormal \(\left(0+x^{2}\right)\), where \({ }^{2}=13^{2}\) is known. Use a nor\(\operatorname{mal}\left(01^{2}\right)\) prior for . What is the posterior distribution of ?
(f) Find a \(95 \%\) credible interval for .
(g) Perform a Bayesian test of
\[
H_{0}:=0 \text { versus } H_{1}:=0
\]
at the \(5 \%\) level of significance.

Transcribed Image Text:

Heart Rate Oxygen Uptake 94 y .47 96 96 .75 94 .83 95 .98 104 1.18 106 1.29 108 1.40 113 1.60 115 1.75 121 1.90 131 2.23