Independent random samples of ceramic produced by two di erent processes were tested for hardness. The results were:

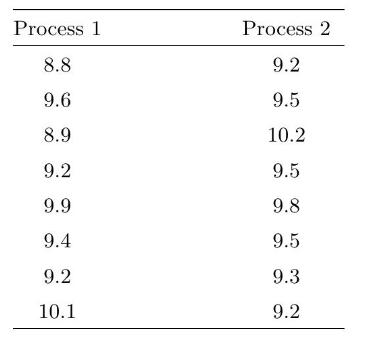
(a) We will assume that the observations come from \(\operatorname{normal}\left({ }_{1}{ }^{2}\right)\) and normal \(\left(2^{2}\right)\), where \({ }^{2}=4^{2}\). Use independent normal \(\left(m s^{2}\right)\) prior

distributions for 1 and \({ }_{2}\), respectively, where \(m=10\) and \(s^{2}=1^{2}\). Find the posterior distributions of \({ }_{1}\) and \({ }_{2}\), respectively.
(b) Find the posterior distribution of \(1 \quad 2\).
(c) Find a \(95 \%\) Bayesian credible interval for \(1 \quad 2\).
(d) Perform a Bayesian test of the hypothesis
\[
\begin{array}{llllllll}
H_{0}: & 1 & 2 & 0 & \text { versus } & H_{1}: & 1 & \\
2
\end{array}\]
at the \(5 \%\) level of signi cance. What conclusion can we draw?

Transcribed Image Text:

Process 1 Process 2 8.8 9.2 9.6 9.5 8.9 10.2 9.2 9.5 9.9 9.8 9.4 9.5 9.2 9.3 10.1 9.2