Consider the helix represented by the vector-valued function r(t) = (2 cos t, 2 sin t, t).

(a) Write the length of the arc s on the helix as a function of t
by evaluating the integral

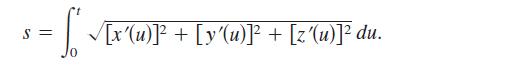
(b) Solve for t in the relationship derived in part (a), and substitute the result into the original set of parametric equations. This yields a parametrization of the curve in terms of the arc length parameter s.

(c) Find the coordinates of the point on the helix for arc lengths s = √5 and s = 4.

(d) Verify that 

Transcribed Image Text:

S= ·[ [x'(u)]²+ [y'(u)]²+ [z'(u)]² du.