Calculate curl(F) and then apply Stokes' Theorem to compute the flux of \(\operatorname{curl}(\mathbf{F})\) through the given surface using a line integral.

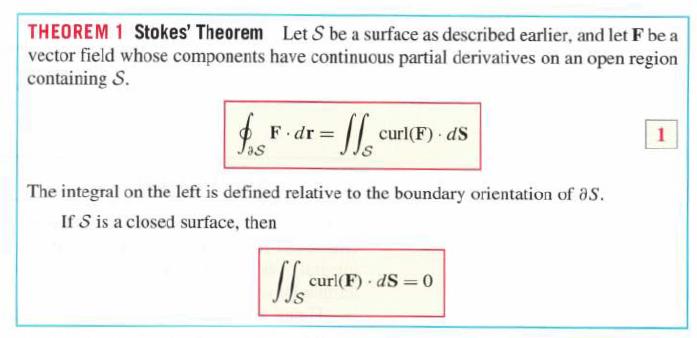
\(\mathbf{F}=\left\langle 2 y, e^{z},-\arctan xightangle\), that part of the paraboloid \(z=4-x^{2}-y^{2}\) cut off by the \(x y\)-plane with upwardpointing unit normal vector

Transcribed Image Text:

THEOREM 1 Stokes' Theorem Let S be a surface as described earlier, and let F be a vector field whose components have continuous partial derivatives on an open region containing S. fos ir = ff curl(F). ds F.dr= The integral on the left is defined relative to the boundary orientation of 85. If S is a closed surface, then 1 curl(F). dS=0 1