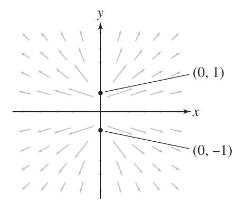
Figure 6 shows the vector field (mathbf{F}=abla f), where [ f(x, y)=ln left(x^{2}+(y-1)^{2}ight)+ln left(x^{2}+(y+1)^{2}ight) ] which is
Question:
Figure 6 shows the vector field \(\mathbf{F}=abla f\), where
\[
f(x, y)=\ln \left(x^{2}+(y-1)^{2}ight)+\ln \left(x^{2}+(y+1)^{2}ight)
\]
which is the velocity field for the flow of a fluid with sources of equal strength at \((0, \pm 1)\) (note that \(f\) is undefined at these two points). Show that \(\mathbf{F}\) is both irrotational and incompressible-that is, \(\operatorname{curl}_{z}(\mathbf{F})=0\) and \(\operatorname{div}(\mathbf{F})=0\) [in computing \(\operatorname{div}(\mathbf{F})\), treat \(\mathbf{F}\) as a vector field in \(\mathbf{R}^{3}\) with a zero \(z\)-component]. Is it necessary to \(\operatorname{compute}_{\operatorname{curl}_{z}(\mathbf{F}) \text { to conclude that it is zero? }}\)
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
To address your question we need to show that the vector field mathbfF abla f is both irrotational and incompressible The vector field mathbfF is give...View the full answer
Answered By
Jaydeep Patidar
As an administrator, I am highly organized, efficient, and able to manage multiple tasks and projects simultaneously. I possess excellent communication and leadership skills and am able to work effectively with staff, colleagues, and clients. I am able to develop and implement policies and procedures to ensure the efficient and effective functioning of an organization. I am also able to manage the budget and resources of an organization, as well as oversee the maintenance and upkeep of facilities.
As a writer, I am able to produce high-quality written materials that are clear, concise, and engaging. I possess strong research skills and am able to write on a wide range of topics. I am able to adapt my writing style to suit the needs of different audiences and am able to work effectively under tight deadlines.
As a project management specialist, I possess a strong understanding of project management methodologies and principles. I am able to plan, organize and execute projects effectively, ensuring that they are completed on time and within budget. I am able to lead and manage cross-functional teams and I am able to identify, track, and mitigate project risks. I am also skilled in using project management software and tools to plan, track and report on project progress. Overall, my combination of skills and experience make me an asset to any organization, and I am confident that I have the ability to excel in any role related to administration, writing, and project management.
0 Reviews
10+ Question Solved
Related Book For 
Question Posted:
