The proof in the text assumes that is increasing. To prove it for all continuous functions,

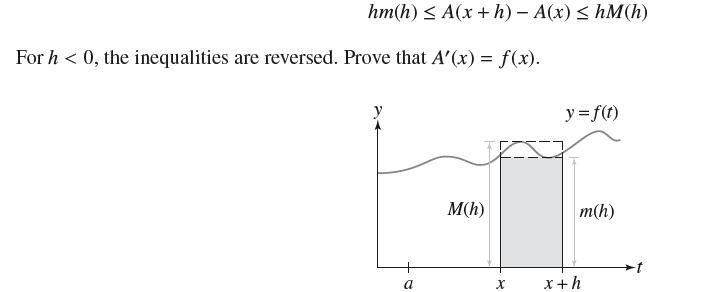
The proof in the text assumes that ƒ is increasing. To prove it for all continuous functions, let m(h) and M(h) denote the minimum and maximum of ƒ on [x, x + h] (Figure 15). The continuity of ƒ implies that M(h) = ƒ(x). Show that for h > 0,

Transcribed Image Text:

lim m(h)= lim M(h) = f(x). h→0 h→0