Use Stokes Theorem to evaluate S curl F ds. F(x, y, z) = x 2
Question:
Use Stokes Theorem to evaluate ∫∫S curl F · ds.
F(x, y, z) = x2y3zi + sin(xyz)j + xyz k, S is the part of the cone y2 = x2 + z2 that lies between the planes y = 0 and y = 3, oriented in the direction of the positive y-axis
Data from Stokes Theorem
Let S be an oriented piecewise-smooth surface that is bounded by a simple, closed, piecewise-smooth boundary curve C with positive orientation. Let F be a vector field whose components have continuous partial derivatives on an open region in R3 that contains S. Then
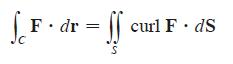
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Related Book For 

Question Posted:





