A particle P moves with constant angular speed around a circle whose center is at the
Question:
A particle P moves with constant angular speed ω around a circle whose center is at the origin and whose radius is R. The particle is said to be in uniform circular motion. Assume that the motion is counterclockwise and that the particle is at the point (R, 0) when t = 0. The position vector at time t ≥ 0 is r(t) = R cos ωt i + R sin ωt j.
(a) Find the velocity vector v and show that v · r = 0. Conclude that v is tangent to the circle and points in the direction of the motion.
(b) Show that the speed |v| of the particle is the constant ωR. The period T of the particle is the time required for one complete revolution. Conclude that
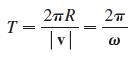
(c) Find the acceleration vector a. Show that it is proportional to r and that it points toward the origin. An acceleration with this property is called a centripetal acceleration. Show that the magnitude of the acceleration vector is |a| = Rω2.
(d) Suppose that the particle has mass m. Show that the magnitude of the force F that is required to produce this motion, called a centripetal force, is
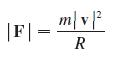
Step by Step Answer:

Calculus Early Transcendentals
ISBN: 9781337613927
9th Edition
Authors: James Stewart, Daniel K. Clegg, Saleem Watson, Lothar Redlin





