The kinetic energy KE of an object of mass m moving with velocity v is defined as KE = 1/2 mv². If a force f(x) acts on the object, moving it along the x-axis from x₁ to x₂, the Work-Energy Theorem states that the net work done is equal to the change in kinetic energy: 1/2 mv²₂ – 1/2 mv²₁, where ν₁ is the velocity at x₁ and ν₂ is the velocity at x₂.

Let x = s(t) be the position function of the object at time t and ν(t), a(t) the velocity and acceleration functions. Prove the Work-Energy Theorem by first using the Substitution Rule for Definite Integrals to show that

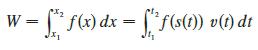
Then use Newton’s Second Law of Motion (force − mass 3 acceleration) and the substitution u = ν(t) to evaluate the integral.

Transcribed Image Text:

) dx = f(s(1)) v(t) dt W =