Complete the following steps to prove that when the x- and y-coordinates of a point on the hyperbola x² - y² = 1 are defined as cosh t and sinh t, respectively, where t is twice the area of the shaded region in the figure, x and y can be expressed as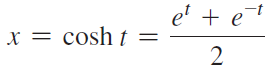and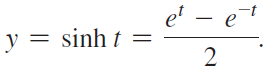

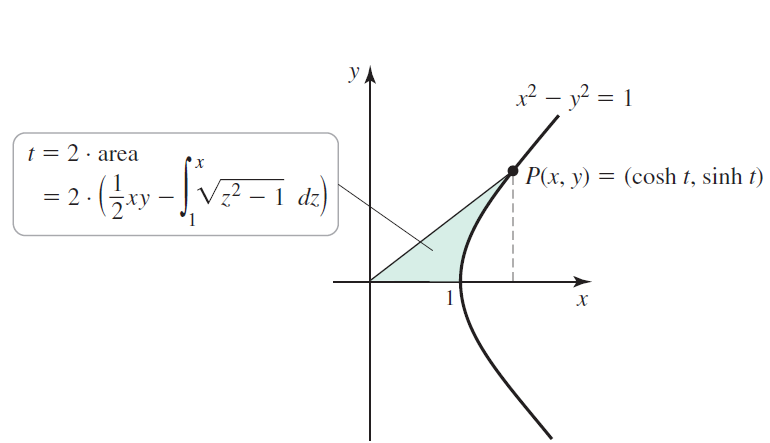
a. Explain why twice the area of the shaded region is given by

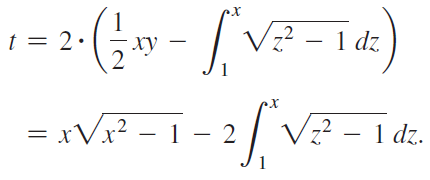
b. The formula for the integral in part (a) is derived:

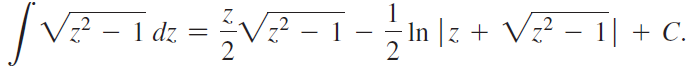
Evaluate this integral on the interval [1, x], explain why the absolute value can be dropped, and combine the result with part (a) to show that

t = ln (x + √x² - 1).

c. Solve the final result from part (b) for x to show that

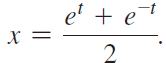
d. Use the fact that y = √x² - 1 in combination with part (c) to show that

Transcribed Image Text:

-t e' + e¯ x = cosh t et y = sinh t et