Consider a pendulum of length L meters swinging only under the influence of gravity. Suppose the pendulum
Question:
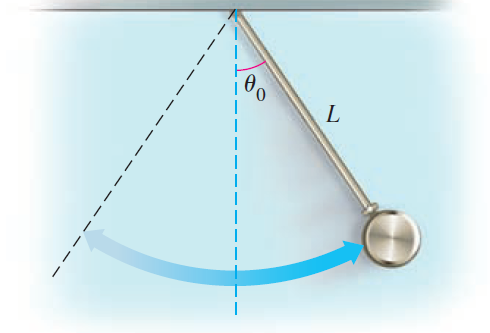
Consider a pendulum of length L meters swinging only under the influence of gravity. Suppose the pendulum starts swinging with an initial displacement of θ0 radians (see figure). The period (time to complete one full cycle) is given by
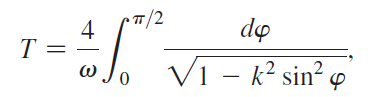
where ω2 = g/L, g ≈ 9.8 m/s2 is the acceleration due to gravity, and k2 = sin2 (θ0/2). Assume L = 9.8 m, which means ω = 1 s-1.
a. Use a computer algebra system to find the period of the pendulum for θ0 = 0.1, 0.2,. . ., 0.9, 1.0 rad.
b. For small values of θ0, the period should be approximately 2π seconds. For what values of θ0 are your computed values within 10% of 2π (relative error less than 0.1)?
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Related Book For 

Calculus Early Transcendentals
ISBN: 978-0321947345
2nd edition
Authors: William L. Briggs, Lyle Cochran, Bernard Gillett
Question Posted:





