Consider the parabola y = x². Let P, Q, and R be points on the parabola with R between P and Q on the curve. Let ℓ_P, ℓ_Q, and ℓ_R be the lines tangent to the parabola at P, Q, and R, respectively (see figure). Let P' be the intersection point of ℓ_Q and ℓ_R, let Q' be the intersection point of ℓ_P and ℓ_R, and let R' be the intersection point of ℓ_P and ℓ_Q. Prove that Area ΔPQR = 2 • Area ΔP'Q'R' in the following cases.

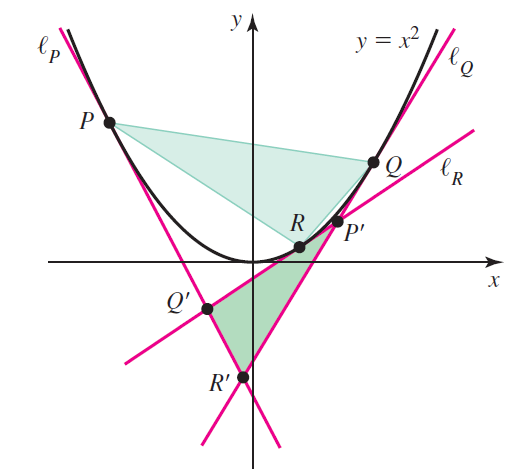
a. P(-a, a²), Q(a, a²), and R(0, 0), where a is a positive real number

b. P(-a, a²), Q(b, b²), and R(0, 0), where a and b are positive real numbers

c. P(-a, a²), Q(b, b²), and R is any point between P and Q on the curve

Transcribed Image Text:

УА y = x2 P' х R'