A thermal swing adsorption process is removing traces of toluene from n-heptane using silica gel adsorbent. Operation is at \(1.0 \mathrm{~atm}\). Feed is \(0.11 \mathrm{wt} \%\) toluene and \(99.89 \mathrm{wt} \% \mathrm{n}-\) heptane at \(0^{\circ} \mathrm{C}\). Feed step is continued until breakthrough occurs. Adsorber is 2.0 meters long and during feed step is at \(0^{\circ} \mathrm{C}\). Purge is counterflow with pure \(\mathrm{n}\)-heptane at \(80^{\circ} \mathrm{C}\). Column is cooled to \(0^{\circ} \mathrm{C}\) before next feed step. Superficial velocity is \(10.0 \mathrm{~cm} / \mathrm{min}\) during both feed and purge steps. Use Eq. (20-15c) for solute velocities. Assume that wall heat capacities can be ignored, heat of adsorption is negligible, and no adsorption of n-heptane. Use solute movement theory to

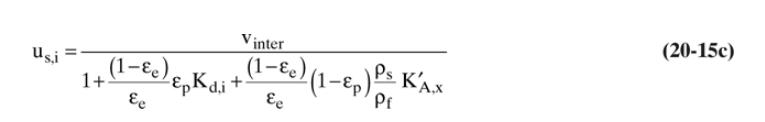
a. determine breakthrough time for toluene during feed step.

b. determine time for thermal wave to break through.

c. determine time to remove all toluene from column.

d. determine outlet concentration profile of toluene in purge fluid.

Data: At low concentrations, isotherms for toluene: \(q=17.46 \times\) at \(0^{\circ} \mathrm{C}, q=7.77 \times\) at \(30^{\circ} \mathrm{C}\), \(\mathrm{q}=5.16 \mathrm{x}\) at \(35^{\circ} \mathrm{C}, \mathrm{q}=1.23 \mathrm{x}\) at \(80^{\circ} \mathrm{C}, \mathrm{q}\) and \(\mathrm{x}\) are in \(\mathrm{g}\) solute/g adsorbent and \(\mathrm{g}\) solute/g fluid (mass fraction), respectively (Matz and Knaebel, 1991). \(ho_{\mathrm{s}}=2100 \mathrm{~kg} / \mathrm{m}^{3}, ho_{\mathrm{f}}=684\) \(\mathrm{kg} / \mathrm{m}^{3}, \mathrm{C}_{\mathrm{ps}}=920 \mathrm{~J} /\left(\mathrm{kg}{ }^{\circ} \mathrm{C}\right), \mathrm{C}_{\mathrm{pf}}=1841 \mathrm{~J} /\left(\mathrm{kg}{ }^{\circ} \mathrm{C}\right), \varepsilon_{\mathrm{e}}=0.43, \varepsilon_{\mathrm{p}}=0.48, \mathrm{~K}_{\mathrm{d}}=1.0\).

Transcribed Image Text:

Us.i Vinter (1-e) & (1-E) (1-e) Ps. KA 1+d,i + Ee Ee Pf (20-15c)