Condensation rates for a binary vapor mixture (adapted from Taylor and Krishna (1993)). Here we apply Eq.
Question:
Condensation rates for a binary vapor mixture (adapted from Taylor and Krishna (1993)). Here we apply Eq. (10.22) to the condensation of a binary mixture of ethylene dichloride (A) and toluene (B) for the following conditions.
Vapor-phase composition: \(y_{\mathrm{A} 0}=0.4\)
Liquid-phase composition at the start of condensation \(x_{\mathrm{A}}=0.325\)
Interface equilibrium relation: \(y=2 x /(1+1.14 x)\)
Vapor-phase mass transfer coefficient under low-flux condition \(0.054 \mathrm{~m} / \mathrm{s}\)
Pressure \(101.325 \mathrm{kPa}\); temperature \(400 \mathrm{~K}\)
Assume that the fluxes are proportional to the liquid composition, i.e., the composition of the first drop is fixed by the relative rates of condensation. This fixes the determinacy condition as
\[x_{\mathrm{A}}=\frac{N_{\mathrm{A}}}{N_{\mathrm{t}}}\]
Use this condition in the relation for \(N_{\mathrm{A}}\) given by Eq. (10.22). Rearrange the equation and show that the total mass flux of condensation is given by \[\begin{equation*}N_{\mathrm{t}}=\ln \left(\frac{x_{\mathrm{AL}}-y_{\mathrm{A} \delta}}{x_{\mathrm{AL}}-y_{\mathrm{A} 0}}\right) \tag{10.92}\end{equation*}\]
Use the numerical values given and verify that \(N_{\mathrm{A}}=0.47\) and \(N_{\mathrm{B}}=0.98\) in the units of \(\mathrm{mol} / \mathrm{m}^{2} \cdot \mathrm{s}\).
Note that in practice the condensation rate is determined not only by the rate of mass transfer but also by the rate at which heat can be removed from the vapor. Hence this is a coupled problem in heat and mass transfer. The example above provides the solution to the mass-transport part of the overall problem.
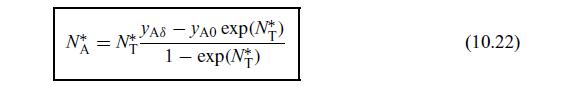
Step by Step Answer:

Advanced Transport Phenomena Analysis Modeling And Computations
ISBN: 9780521762618
1st Edition
Authors: P. A. Ramachandran





