Repeat Problem 5.H1, but with a boilup ratio of 2.5. After solving Problem 5.H1, H3 should be quite straightforward.

Problem 5.H1

Do the stage-by-stage calculations for Problem 5.D14. Determine the number of stages required and the mole fractions of the components in the vapor distillate. Either develop your own spreadsheet or modify the spreadsheet in Figure 5A-1 and Table 5A-2. To modify, first change the notation to separate benzene, toluene, and xylene and change the input variables to those calculated in Problem 5.D14. Then replace rows 3 to 6 with the constant relativity values for benzene, toluene, and xylene in column B. Next, delete the formulas in columns B, C, and D for rows 22, 23, and 24. In B22, B23, and B24, write the formulae to calculate \(\mathrm{x}_{\text {benzene }}, \mathrm{x}_{\text {toluene }}\), and \(\mathrm{x}_{\mathrm{xylenes}}\) using Eq. (5-28). The yop values for the three components can be calculated from the formulae in F22, F23, and F24. Record the yop values in a separate table. For the next stage, manually transfer the yop values from the saved table to cells B17, D17, and F17.

Problem 5.D14.

We are separating a mixture of benzene, toluene, and xylene in a distillation stripping column that has a partial reboiler and no condenser. The feed is a saturated liquid, \(\mathrm{F}=135 \mathrm{kmol} / \mathrm{h}\), and feed is \(18.5 \mathrm{~mol} \%\) benzene, \(30.0 \mathrm{~mol} \%\) toluene, and remainder xylenes. Pressure is \(1.0 \mathrm{~atm}, \mathrm{CMO}\) is valid, and the relative volatilities are constant: \(\alpha_{\text {ben-tol }}=2.5, \alpha_{\text {tol-xy }}=3.030\). The boilup ratio »/B \(=5\), and the bottoms is 0.98 or higher mole fraction xylene. See problem 5.C1 for the method of calculating equilibrium with constant relative volatilities.

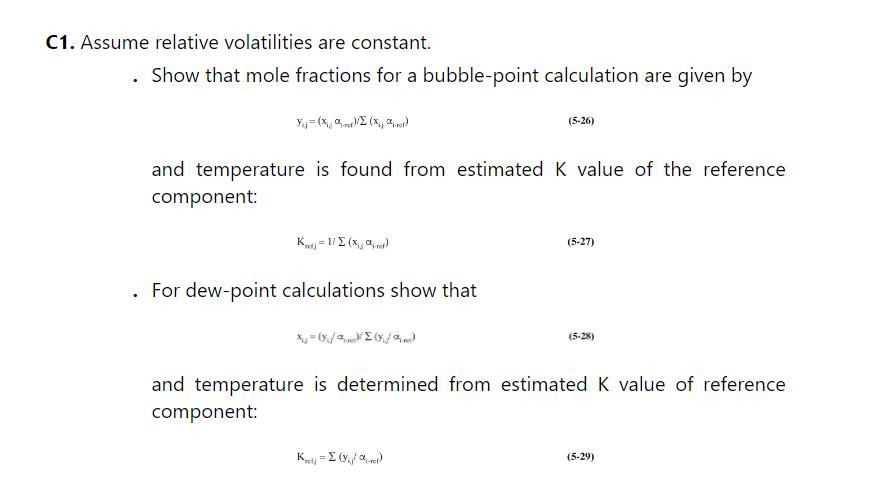
a. Based on the best assumption you can make, calculate B, mole fractions in bottoms, \(\mathrm{D}\), mole fractions in vapor distillate and \(\pi / \mathrm{r}\).

b. Show the calculation to determine mole fractions in liquid from the top stage (\#1) that are in equilibrium with the vapor distillate.

c. Show the calculation to determine mole fractions in vapor leaving stage 2. Stage-by-stage calculations are continued in problem 5.H1.

Transcribed Image Text:

C1. Assume relative volatilities are constant. Show that mole fractions for a bubble-point calculation are given by =(x)/(x) (5-26) and temperature is found from estimated K value of the reference component: K=1/(x) . For dew-point calculations show that (5-27) x-(%) (5-28) and temperature is determined from estimated K value of reference component: = Kret(y) (5-29)